Spin-Based Quantum Computing
Over the last decade the field of quantum computation has seen spectacular progress not only at the theoretical level but also within experimental physics, chemistry and materials science. It is now believed that essential quantum mechanical properties such as quantum entanglement and superposition can be considered as fundamental natural resources, of comparable importance to energy, information or entropy. Apart from the early developments of quantum algorithms and their remarkable ability in solving certain computational tasks, there is currently an ever increasing interest in finding appropriate physical systems to implement key concepts of quantum computation which reflects the emerging challenges of faithfully storing, processing, and measuring quantum information. To this end, electron and nuclear spins are considered promising quantum information (qubits) elements because they are natural two-(or higher) state systems with relatively long decoherence times that can be controlled using well-established magnetic resonance techniques.
Electron Spin Coherence of Atomic Hydrogen encapsulated in POSS cages
Encapsulated atomic hydrogen in polyhedral oligomeric silsesquioxane (POSS) cages

is a promising candidate for applications in emerging technologies like spin-based quantum computing, magnetic field sensing, and atomic clock devices. Compared to endohedral fullerenes (N@C60 or P@C60), which are currently the most used molecular spin systems for demonstrating single-quantum gates and quantum memories, atomic hydrogen is more attractive due to its simpler electronic 1s state and the exceptionally large hyperfine coupling of about 1420 MHz. Detailed pulsed EPR studies of parameters relevant to quantum computing like electron spin-lattice (T1) and phase memory (TM) relaxation times are scarce and concern exclusively cages of the type Si8O12R8 with R = C2H5, R = C3H7 (n-propyl), and R = OSi(CH3)2H. Recently, we applied dynamical decoupling methods in order to suppress nuclear spin diffusion in H@h72Q8M8, the derivative with R = OSi(CH3)3. Moreover, we examined for the first time the effect of deuterium isotopic substitution on the spin relaxation properties of H@h72Q8M8, by applying pulsed electron paramagnetic resonance (EPR) methods on its deuterated analogues H@d72Q8M8 and D@d72Q8M8. For the latter species we measured a phase memory time of 60 μs at 180 K, the largest obtained so far for this family of molecular spins, however, we observed a persistent shortening of TM even at liquid helium temperatures. Herein we shed light into this mechanism of decoherence, assumed to be tunneling reorientation of methyl groups, by studying POSS cages with modified organic substituents R. As a first example we present spin relaxation measurements on a derivative with R = CH=CH2 (vinyl) containing an organic group with different rotational degrees of freedom compared to the previoulsly studied R = OSi(CH3)3. We determine the activation energy of this new rotational mode and we find a modest effect on electron spin coherence as obtained from the considerably different temperature dependence of TM.
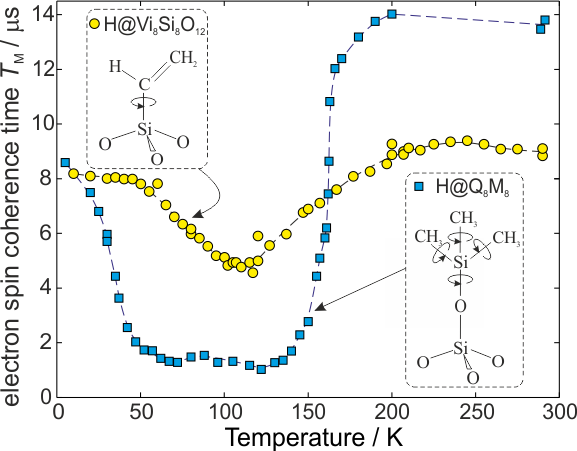
Parallel-mode EPR on Atomic Hydrogen encapsulated in POSS cages
The successful synthesis of H@h72Q8M8, and its deuterated analogues D@d72Q8M8 and H@d72Q8M8, triggered an unconventional study using the so-called parallel-mode EPR.

Parallel-mode EPR, where the oscillating microwave magnetic field B1 is parallel to the static field B0, is mostly used in biologically relevant studies as they often involve high spin systems. Although such approach may seem meaningless for S = 1∕2 systems, measurement in the parallel-mode can also give non-zero EPR spectra for hyperfine-coupled S = 1∕2 systems provided that there is strong mixing of electron (MS) and nuclear (MI) spin states, so that MS is not a good quantum number. A sufficient mixing of states requires that the electron Zeeman and hyperfine interaction terms have comparable strengths, a condition that is met for low-field EPR spectroscopy and/or systems with exceptionally strong hyperfine coupling constants. The hydrogen atom (1H) is the most typical example of an electronic spin S = 1∕2 , hyperfine-coupled to a proton nuclear spin, I = 1∕2 , with a large isotropic coupling constant of A0∕h = 1420.40575 MHz. Recently, we reported the first dual-mode X-band EPR experiments of hydrogen atom (both isotopes H and D) encapsulated in POSS cages. Firstly we extended the theory to the system of an electron spin S = 1∕2 coupled to a nuclear spin I = 1, and we derived analytical expressions for the resonance fields and the transition probabilities both for perpendicular and parallel modes. We then examined some interesting features of the forbidden transitions like their first-order dependence on the nuclear g-factor, gn , and the existence of a clock transition with f = 307 MHz that in the future can be exploited in portable atomic clock devices.

Hybrid electron-nuclear spin systems
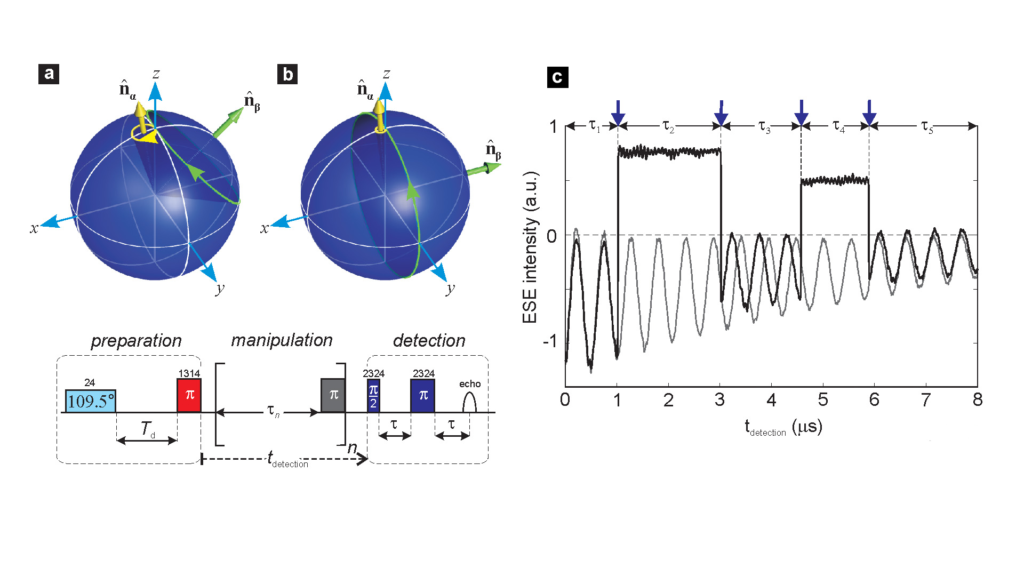
Nuclear and electron spins are ideal elements for quantum computation (qubits: quantum bits) because they are natural two-state systems and decoherence times for the spin degree of freedom are usually larger than for charge degrees of freedom. A fundamental challenge in the realization of a solid-state quantum computer is the construction of fast and reliable two-qubit quantum gates. Of particular interest in this direction are hybrid systems of electron and nuclear spins, where the two qubits are coupled through the hyperfine interaction (hfi). However, the significantly different decoherence times of electron (T2e) and nuclear (T2n) spins do not allow for their coherent manipulation at the same time scale. Moreover, the combination of the small gyromagnetic ratios gn of nuclei with the currently available rf fields B1, results in very slow rotations of nuclear spins. For instance, the nutation (Rabi) frequency of a proton nuclear spin for a typical value of B1=1 mT is ω1/2π = gnB1/h = 42.6 kHz which implies a length of Δtπ = 11.7 ms for an rf π-pulse. This time interval is of the same order of magnitude with typical electron decoherence times, T2e, and thus the efficiency of the two-qubit quantum gate may become questionable due to relaxation losses. One way to overcome this difficulty is to utilize the hyperfine interaction which is typically stronger than the Rabi nuclear frequency, i.e. of the order of some MHz in organic radicals. When the hfi is anisotropic the quantization axes of the hyperfine fields deviate from the z axis (Fig. a). Here we consider a special case of ESEEM spectroscopy, the so-called exact cancellation, where the hfi and electron Zeeman interaction cancel each other in one mS manifold. Under this condition, the effective magnetic field at the nucleus is either almost parallel or exactly perpendicular to the z axis, depending on the state of the electron spin (Fig. b). By using a single crystal of γ-irradiated malonic acid, we demonstrated how this method overcomes the problem of relaxation incompatibility between electron and nuclear spin qubits. The nutation period for the proton nuclear spin is only 250 ns, about 50 times shorter than 11.7 ms which is required for an rf π-pulse (Fig. c). No rf irradiation is needed and therefore, the method is free from artifacts related to rf B1 inhomogeneity.
References
- Spin Quantum Computing with Molecular-Encaged Atomic Hydrogen, George Mitrikas, ERCIM NEWS 128, 43-44 (2022)
- Electron Spin Relaxation Mechanisms of Atomic Hydrogen Trapped in Silsesquioxane Cages: the Role of Isotope Substitution, George Mitrikas and Raanan Carmieli, J. Phys. Chem. C 125, 9899−9907 (2021) DOI: 10.1021/acs.jpcc.1c01582
- Parallel‑Mode EPR of Atomic Hydrogen Encapsulated in POSS Cages, George Mitrikas, Yiannis Sanakis, Nikolaos Ioannidis, Applied Magnetic Resonance 51, 1451–1466 (2020) DOI: 10.1007/s00723-020-01263-5
- Electron spin relaxation properties of atomic hydrogen encapsulated in octavinyl POSS cages, George Mitrikas and Stavroula Menenakou, Phys. Chem. Chem. Phys. 22, 15751-15758 (2020) DOI: 10.1039/d0cp02479a
- Extending the electron spin coherence time of atomic hydrogen by dynamical decoupling, G. Mitrikas, E. K. Efthimiadou, G. Kordas, Phys. Chem. Chem. Phys. 16, 2378-2383 (2014) DOI: 10.1039/c3cp53423e
- Pulsed EPR characterization of encapsulated atomic hydrogen in octasilsesquioxane cages, George Mitrikas, Phys. Chem. Chem. Phys. 14, 3782-3790 (2012) DOI: 10.1039/c2cp24057b
- Ultrafast control of nuclear spins using only microwave pulses: Towards switchable solid-state quantum gates, George Mitrikas, Yiannis Sanakis, and Georgios Papavassiliou, Phys. Rev. A 81, 020305(R) (2010) DOI: 10.1103/PhysRevA.81.020305
- Electron spin-lattice and spin-spin relaxation study of a trinuclear iron (III) complex and its relevance in quantum computing, George Mitrikas, Yiannis Sanakis, Catherine P. Raptopoulou, George Kordas and Georgios Papavassiliou, Phys. Chem. Chem. Phys. 10, 743-748 (2008) DOI: 10.1039/b711056a